5 Ways to Understand Riemann Surfaces of Finite Type

What are Riemann Surfaces?
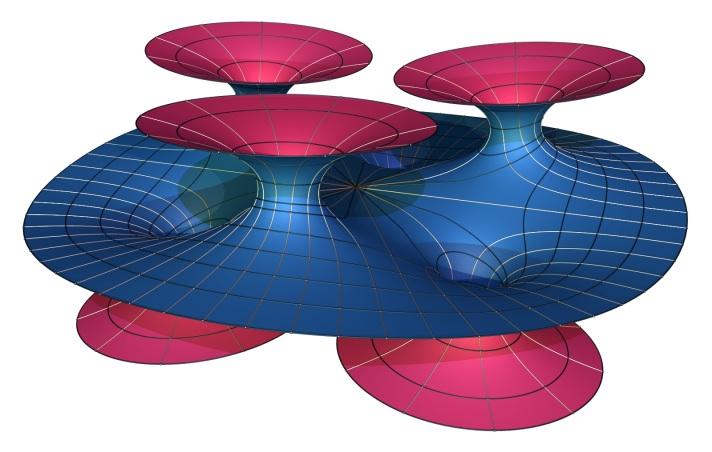
Riemann surfaces are a fundamental concept in mathematics, specifically in the field of algebraic geometry and complex analysis. They are named after the German mathematician Bernhard Riemann, who first introduced them in the 19th century. In essence, a Riemann surface is a one-dimensional complex manifold, which means it is a space that is locally like the complex plane, but may have a more complicated global structure.
Finite Type Riemann Surfaces

A Riemann surface of finite type is a Riemann surface that can be compactified by adding a finite number of points, called punctures. This means that the surface can be made compact by adding a finite number of points at infinity. Riemann surfaces of finite type are important in mathematics because they have many interesting properties and are used to study various problems in algebraic geometry, number theory, and theoretical physics.
1. Genus and Topology

One way to understand Riemann surfaces of finite type is through their topology. The genus of a Riemann surface is a topological invariant that measures the number of “holes” in the surface. For example, a sphere has genus 0, a torus has genus 1, and a surface with two holes has genus 2. Riemann surfaces of finite type have a finite genus, which means that they have a finite number of holes.
2. Algebraic Curves

Another way to understand Riemann surfaces of finite type is through algebraic curves. An algebraic curve is a curve defined by a polynomial equation in two variables. Riemann surfaces of finite type can be obtained by compactifying algebraic curves. For example, the curve defined by the equation y^2 = x^3 - x has a Riemann surface of genus 1, which is a torus.
3. Moduli Spaces

Moduli spaces are spaces that parameterize Riemann surfaces of a given type. For example, the moduli space of genus 1 Riemann surfaces is the space of all possible tori. Moduli spaces are important in mathematics because they provide a way to study the properties of Riemann surfaces in a systematic way. Riemann surfaces of finite type have moduli spaces that are finite-dimensional, which means that they can be parameterized by a finite number of parameters.
4. Teichmüller Spaces

Teichmüller spaces are spaces that parameterize Riemann surfaces of a given type, up to a certain equivalence relation. For example, the Teichmüller space of genus 1 Riemann surfaces is the space of all possible tori, up to a certain equivalence relation. Teichmüller spaces are important in mathematics because they provide a way to study the properties of Riemann surfaces in a more detailed way. Riemann surfaces of finite type have Teichmüller spaces that are finite-dimensional, which means that they can be parameterized by a finite number of parameters.
5. Uniformization Theorem
The uniformization theorem is a fundamental result in mathematics that states that every Riemann surface can be uniformized, meaning that it can be represented as a quotient of the upper half-plane or the unit disk by a group of transformations. Riemann surfaces of finite type can be uniformized by a finite group of transformations, which means that they can be represented as a quotient of the upper half-plane or the unit disk by a finite group.
💡 Note: The uniformization theorem is a powerful tool for studying Riemann surfaces, but it requires a good understanding of complex analysis and group theory.
| Method | Description |
|---|---|
| Genus and Topology | Studies the topological properties of Riemann surfaces, such as the number of holes. |
| Algebraic Curves | Studies the algebraic properties of Riemann surfaces, such as the polynomial equations that define them. |
| Moduli Spaces | Studies the spaces that parameterize Riemann surfaces of a given type. |
| Teichmüller Spaces | Studies the spaces that parameterize Riemann surfaces of a given type, up to a certain equivalence relation. |
| Uniformization Theorem | Represents Riemann surfaces as a quotient of the upper half-plane or the unit disk by a group of transformations. |

Riemann surfaces of finite type are a fascinating area of mathematics that have many interesting properties and applications. By understanding these surfaces through different methods, such as genus and topology, algebraic curves, moduli spaces, Teichmüller spaces, and the uniformization theorem, we can gain a deeper insight into the nature of these surfaces and their role in mathematics.
What is a Riemann surface?

+
A Riemann surface is a one-dimensional complex manifold, which means it is a space that is locally like the complex plane, but may have a more complicated global structure.
What is a Riemann surface of finite type?
+
A Riemann surface of finite type is a Riemann surface that can be compactified by adding a finite number of points, called punctures.
What is the uniformization theorem?

+
The uniformization theorem is a fundamental result in mathematics that states that every Riemann surface can be uniformized, meaning that it can be represented as a quotient of the upper half-plane or the unit disk by a group of transformations.



